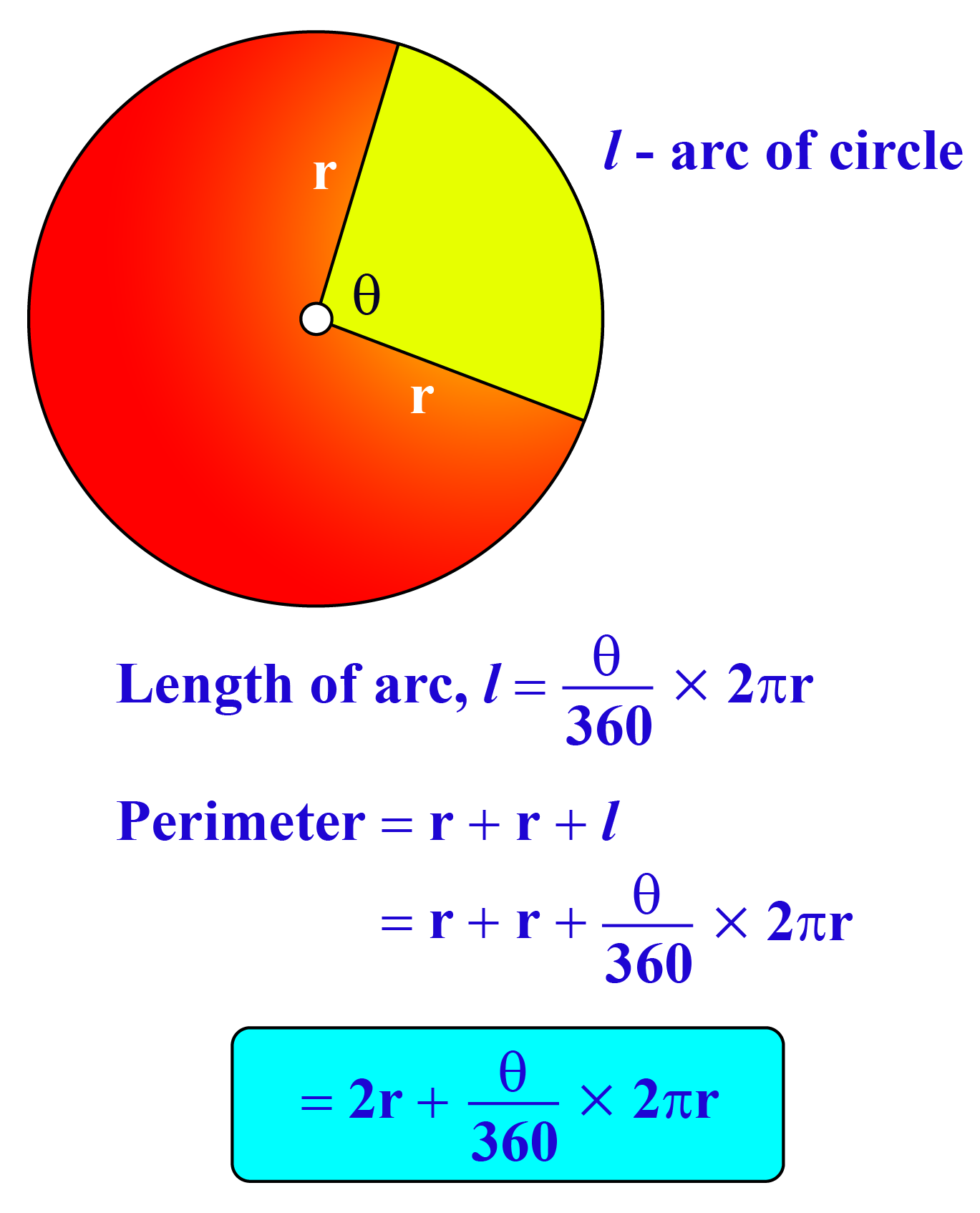
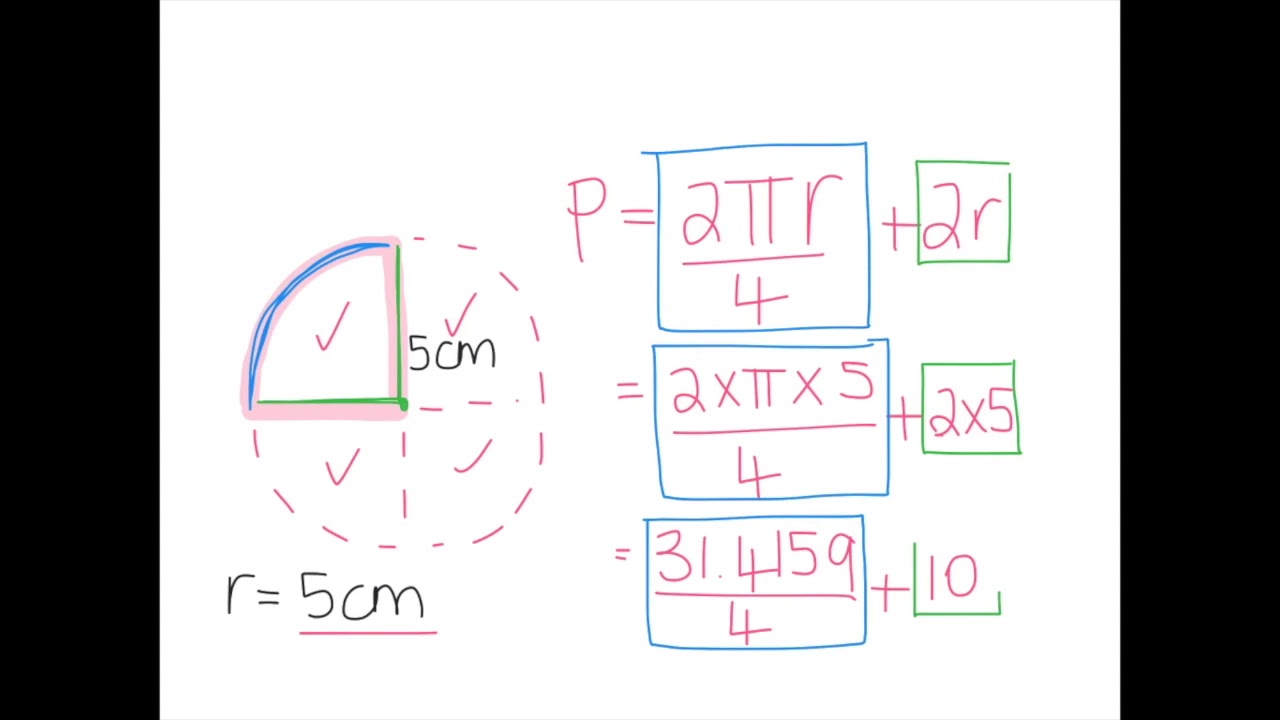
Calculating Area Of A Circle Using Circumference

Calculating areas and circumferences of circles plays an important role in almost all field of science and real life. For instance, formula for circumference and area of a circle can be applied into geometry. They are used to explore many other formulas and mathematical equations. An arch length is a portion of the circumference of a circle. The ratio of the length of an arc to the circumference is equal to the ratio of the measure of the arc to $360$ degrees.

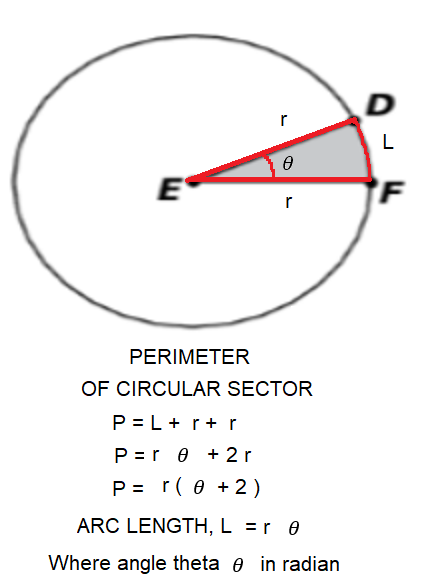
A sector of a circles is the region bounded by two radii of the circle and their intercepted arc. As with triangles and rectangles, we can attempt to derive formulas for the area and "perimeter" of a circle. Calculating the circumference of a circle is not as easy as calculating the perimeter of a rectangle or triangle, however.

This first argument is an example of MP7, Look For and Make Use of Structure. The key to this argument is identifying that all circles are similar and then applying the meaning of similarity to the circumference. The second argument exemplifies MP8, Look For and Express Regularity in Repeated Reasoning.

Here the key is to compare the circle to a more familiar shape, the triangle. The area of the circle can be conveniently calculated either from the radius, diameter, or circumference of the circle. The constant used in the calculation of the area of a circle is pi, and it has a fractional numeric value of 22/7 or a decimal value of 3.14.

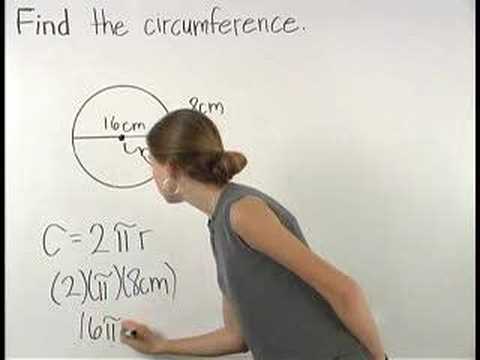
Any of the values of pi can be used based on the requirement and the need of the equations. The below table shows the list of formulae if we know the radius, the diameter, or the circumference of a circle. The circumference of a circle of radius $r$ is $2\pi r$. This well known formula is taken up here from the point of view of similarity. It is important to note in this task that the definition of $\pi$ already involves the circumference of a circle, a particular circle. In order to show that the ratio of circumference to diameter does not depend on the size of the circle, a similarity argument is required.

Two different approaches are provided, one using the fact that all circles are similar and a second using similar triangles. This former approach is simpler but the latter has the advantage of leading into an argument for calculating the area of a circle. Circle is a particular shape and defined as the set of points in a plane placed at equal distance from a single point called the center of the circle.

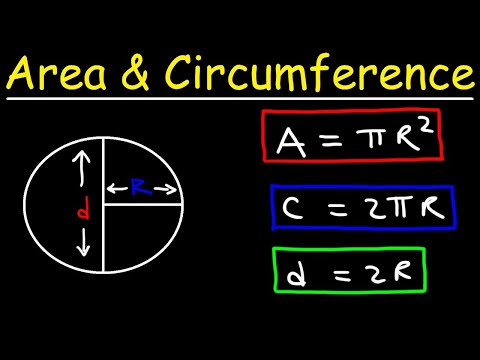
We use the circle formula to calculate the area, diameter, and circumference of a circle. Radius is half the length of a diameter of the circle. Area of the circle describes the amount of space covered by the circle and the length of the boundary of the circle is known as its circumference.

For those having difficulty using formulas manually to find the area, circumference, radius and diameter of a circle, this circle calculator is just for you. The equations will be given below so you can see how the calculator obtains the values, but all you have to do is input the basic information. A circle can be divided into many small sectors which can then be rearranged accordingly to form a parallelogram. When the circle is divided into even smaller sectors, it gradually becomes the shape of a rectangle. We can clearly see that one of the sides of the rectangle will be the radius and the other will be half the length of the circumference, i.e, π.

As we know that the area of a rectangle is its length multiplied by the breadth which is π multiplied to 'r'. Is called the circumference and is the linear distance around the edge of a circle. The circumference of a circle is proportional to its diameter, d, and its radius, r, and relates to the famous mathematical constant, pi (π). The properties of circles have been studied for over 2,000[/latex] years.

All circles have exactly the same shape, but their sizes are affected by the length of the radius, a line segment from the center to any point on the circle. A line segment that passes through a circle's center connecting two points on the circle is called a diameter. The first solution requires a general understanding of similarity of shapes while the second requires knowledge of similarity specific to triangles. This concept can be of significance in geometry, to find the perimeter, area and volume of solids. Real life problems on circles involving arc length, sector of a circle, area and circumference are very common, so this concept can be of great importance of solving problems.

When you are doing calculations involving a circle, you frequently use the number π, or pi. Pi is defined as being equal to the circumference of a circle -- the distance around that circle -- divided by its diameter. However, you don't need to memorize this formula when working with π, since it is a constant. The perimeter and area of triangles, quadrilaterals , circles, arcs, sectors and composite shapes can all be calculated using relevant formulae.

From point B, on the circle, draw another circle with center at B, and radius OB. The intersections of the two circles at A and E form equilateral triangles AOB and EOB, since they are composed of 3 congruent radii. Extend the radii forming these triangles through circle O to form the inscribed regular hexagon with 6 equilateral triangles. A circle is a closed curve formed by a set of points on a plane that are the same distance from its center. The area of a circle is the region enclosed by the circle. The area of a circle is equals to pi (π) multiplied by its radius squared.

Finding the radius is not always easy, especially if you don't have the circle's center. You can calculate the area using the diameter instead. The same formula applies as above, but you need first to calculate the radius of the circle. Simply divide the diameter by 2 to get the radius. Simply enter the desired value in the relevant box. Please use only numbers (e.g. enter 22 not 22 cm).

If you try to enter a unit of measure (e.g. 22 metres, 4 miles, 10 cm) you will get an NAN error appear in each box. When you have entered the number that you know, click the button on the right of that box to calculate all the other values. For example, if you know the volume of a sphere enter the value into the bottom box and then click the calculate button at bottom right. The diameter of a circle is twice to that of the radius. If the diameter or radius of a circle is given, then we can easily find the circumference.

We can also find the diameter and radius of a circle if the circumference is given. We round off to 3.14 in order to simplify our calculations. Circumference, diameter and radii are calculated in linear units, such as inches and centimeters. A circle has many different radii and many different diameters, and each one passes through the center. For any other value for the length of the radius of a circle, just supply a positive real number and click on the GENERATE WORK button. They can use these methods in order to determine the area and lengths of parts of a circle.

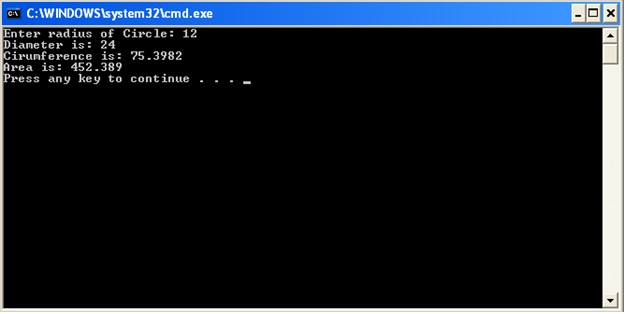
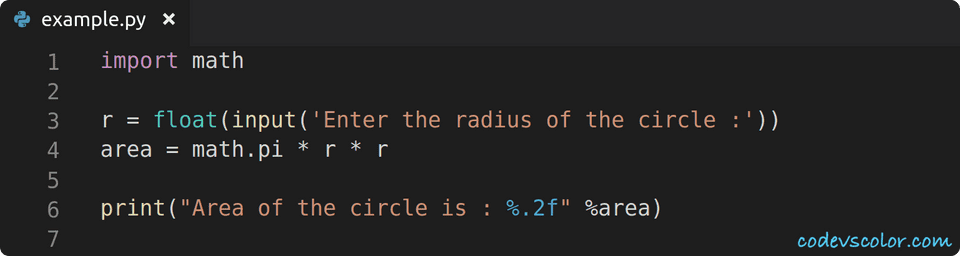
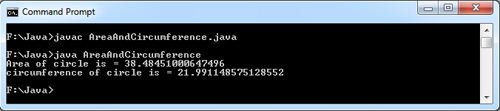
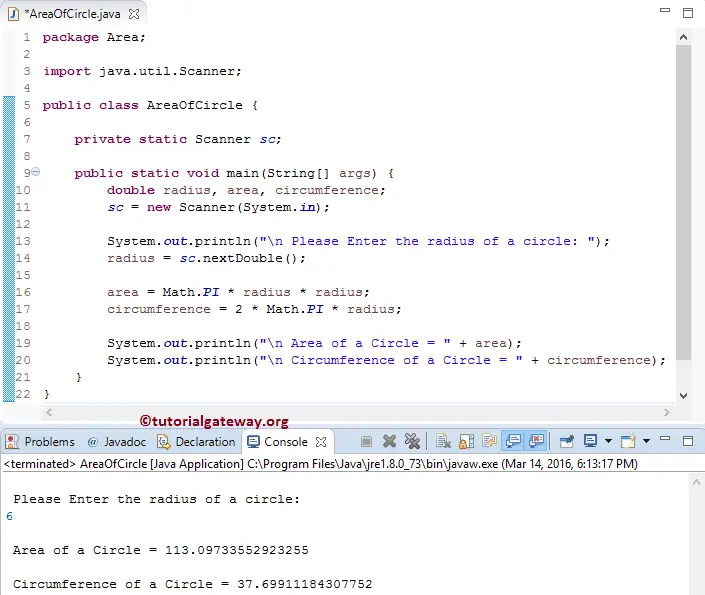
In above program, we first take radius of circle as input from user and store it in variable radius. Then we calculate the circumference of circle using above mentioned formulae and print it on screen using cout. Calculating either the circumference or area of a circle requires knowing the circle's radius. A circle's radius is the distance from the center of the circle to any point on the edge of the circle. Radius is the same for all points on a circle's edge. One of your problems might give you diameter instead of radius and ask you to solve for area or circumference.

A circle's diameter is equal to the distance across the center of the circle, and is equal to the radius times 2. So, you can convert diameter to radius by dividing the diameter by 2. For example, a circle with a diameter of 8 has a radius of 4. A circle is a collection of points that are at a fixed distance from the center of the circle. We see circles in everyday life such as a wheel, pizzas, a circular ground, etc.

The measure of the space or region enclosed inside the circle is known as the area of the circle. The area of a circle formula is useful for measuring the region occupied by a circular field or a plot. Suppose, if you have a circular table, then the area formula will help us to know how much cloth is needed to cover it completely.

The area formula will also help us to know the boundary length i.e., the circumference of the circle. A circle is a two-dimensional shape, it does not have volume. A circle only has an area and perimeter/circumference. Let us learn in detail about the area of a circle, surface area, and its circumference with examples.

The sectors are pulled out of the circle and are arranged as shown in the middle diagram. The length across the top is half of the circumference. When placed in these positions, the sectors form a parallelogram. The larger the number of sectors that are cut, the less curvy the arcs will appear and the more the shape will resemble a parallelogram.

As seen in the last diagram, the parallelogram ca be changed into a rectangle by slicing half of the last sector and placing it to the far left. "R" is used to represent the radius of the circle. It is the distance of any line from the center of the circle to the circle's edge. You can also calculate the radius by dividing the diameter by 2. Only a mathematician can genuinely understand the practical importance of formulas for calculating area, radius, diameter, or circle circumference.

While most people think that formulas have no practical use, they are critical factors in many everyday life routines. To understand how to calculate circumference we must first begin with the definition of circumference. Circumference of a circle is linear distance around outer border of a circle. To find out the circumference, we need to know its diameter which is the length of its widest part. The diameter should be measured in feet for square footage calculations and if needed, converted to inches , yards , centimetres , millimetres and metres . A set of points in a plane equally distanced from a given point $O$ is a circle.

The point $O$ is called the center of the circle. The distance from the center of a circle to any point on the circle is called the radius of this circle.A radius of a circle must be a positive real number. The circle with a center $O$ and a radius $r$ is denoted by $c$. Today I am going to explain how to calculate the area and circumference of a circle using a class in TypeScript. Using this we can perform other types of calculations and generate the results. Lauren is planning her trip to London, and she wants to take a ride on the famous ferris wheel called the London Eye.

While researching facts about the giant ferris wheel, she learns that the radius of the circle measures approximately 68 meters. What is the approximate circumference of the ferris wheel? Say you're trying to calculate the area of a circle with a radius of 3 inches.

You would multiply 3 times 3 to get 9, and multiply 9 times π. Also note that when you multiply inches by inches, you get square inches, which is a measurement of area instead of length. The circle's circumference — the measure of the total length around the shape — is determined based on the fixed ratio of Pi. In degrees, a circle is equal to 360° and Pi is the fixed ratio equal to 3.14.

The o is the center point of the circle, and r is the radius. Now the circle's circumference or perimeter would be precisely the rope's length that wraps around the circle. In this method, we divide the circle into 16 equal sectors.

The sectors are arranged in such a way that they form a rectangle. All sectors are similar in area, so hence all sectors' arc length would be equal. The circle's area would be the same as the area of the parallelogram shape or rectangle.

The area of a circle is any space that the circle occupies on a flat surface. When we talk about the surface area of the circle, we are focusing on two-dimensional objects. When finding the circle area, there are three other measures that we take into consideration, including the circumference, diameter, and radius. All three calculations also help us fining the circle area. Not just this but there are some significant distances on a circle that needs to be calculated before finding the circumference of the circle.

Diameter is the distance from one side of the circle to the other, crossing through the center/ middle of the circle. I am going to discuss these axioms in a moment, but first let me show how Claim follows. But by Axiom 1 the length of the arc $PBQ$ is greater than $PQ$, while it follows from Euclid III.2 that $OB$ is greater than $OA$.

Applying symmetry, this implies Claim in this case, and ought to suggest how the reasoning goes in general. Area and circumference of circle calculator uses radius length of a circle, and calculates the perimeter and area of the circle. It is an online Geometry tool requires radius length of a circle.